Join Us On Social Media!
Italy
Recent Posts
📝 Question of the Day?
3 hours ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 8 Views · 0 Likes
Flag
📝 Reply
I think this question was very easy for the English, or at least for those familiar with their country's naval history.
I had read about this particular episode in two contexts.
One was during the Seven Years' War, and another (I've attached some images, including the one referring to Bing) regarding discipline aboard 18th-century ships, particularly British ships.
It may seem strange, but it was indeed true, and it's one of the articles in the larger section dedicated to life at sea.
I believe they were extremely severe with Bing.
In other cases, both older and more recent, this wasn't the case.
I had read about this particular episode in two contexts.
One was during the Seven Years' War, and another (I've attached some images, including the one referring to Bing) regarding discipline aboard 18th-century ships, particularly British ships.
It may seem strange, but it was indeed true, and it's one of the articles in the larger section dedicated to life at sea.
I believe they were extremely severe with Bing.
In other cases, both older and more recent, this wasn't the case.
You need to answer todays 'Question of the Day' before viewing this post!
(Alternatively, this post will be made visible tomorrow)
(Alternatively, this post will be made visible tomorrow)
▲
⟩⟩
No likes yet
This member will receive 1 point
for every like received
This member will receive 1 point
for every like received
Login To
Remove Ads
Remove Ads
💬 Re: And...... another Titanic
20 hours ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 30 Views · 3 Likes
Flag
💬 Add Comment
Beautiful navigation and beautiful model.
▲
⟩⟩
hermank
Nickthesteam
DuncanP
💬 Re: Re: Re: The Hood
23 hours ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 0 Views · 0 Likes
Flag
💬 Add Comment
Beautiful video, congratulations, Sir Kay.
Of course, it's much harder to make the video realistic, because the proportion of the waves to the ship is obvious.
In many of your photos, it's hard to tell that it's a model and not a real ship.
I think you're one of the best at this.
I've marked myself as your follower.
Of course, it's much harder to make the video realistic, because the proportion of the waves to the ship is obvious.
In many of your photos, it's hard to tell that it's a model and not a real ship.
I think you're one of the best at this.
I've marked myself as your follower.
▲
⟩⟩
No likes yet
This member will receive 1 point
for every like received
This member will receive 1 point
for every like received
💬 Re: Sir Geraint
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 15 Views · 2 Likes
Flag
💬 Add Comment
Sir Ray, as I wrote in my review of the St Canute model, these fantastic photos enhance an already beautiful boat.
The last photo is exceptional; it almost looks like the real thing, not a scale model. Three other images also look like real boats. Well done.
The last photo is exceptional; it almost looks like the real thing, not a scale model. Three other images also look like real boats. Well done.
▲
⟩⟩
DuncanP
Wolle
💬 Re: Another St Canute
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 14 Views · 1 Like
Flag
💬 Add Comment
Sir Ray, these beautiful photos really enhance an already very beautiful scale naval model.
▲
⟩⟩
DWBrinkman
💬 Re: Prince of Wales
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 17 Views · 0 Likes
Flag
💬 Add Comment
Beautiful model.
I also enjoyed the navigation even though the video was very short.
I also enjoyed the navigation even though the video was very short.
▲
⟩⟩
No likes yet
This member will receive 1 point
for every like received
This member will receive 1 point
for every like received
💬 Re: Bluejacket Cape Cod Catboat
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 9 Views · 0 Likes
Flag
💬 Add Comment
Hi Sir Kay, beautiful model cat, I would have liked to see it sailing but it still looks good on static display.
▲
⟩⟩
No likes yet
This member will receive 1 point
for every like received
This member will receive 1 point
for every like received
💬 Re: The Hood
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 11 Views · 0 Likes · 1 Comment
Flag
💬 Add Comment
Beautiful photos and a beautiful model. I'm a little late in commenting, but I only discovered your harbor after you commented on one of my threads. Congratulations.
How long is your model?
I think it's 1:350 scale, so it should be about 75 cm (0.75 m).
Do you have any videos on YouTube? I'd love to see it underway.
How long is your model?
I think it's 1:350 scale, so it should be about 75 cm (0.75 m).
Do you have any videos on YouTube? I'd love to see it underway.
▲
⟩⟩
No likes yet
This member will receive 1 point
for every like received
This member will receive 1 point
for every like received
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 14 Views · 0 Likes
Flag
📝 Reply
Thanks so much Jock, I'm really glad you like it.
▲
⟩⟩
No likes yet
This member will receive 1 point
for every like received
This member will receive 1 point
for every like received
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 16 Views · 1 Like
Flag
📝 Reply
Hi Mike, I haven't forgotten about you.
I couldn't write right away.
Thank you so much for your appreciation and kind words.
I couldn't write right away.
Thank you so much for your appreciation and kind words.
▲
⟩⟩
hermank
📝 Animal and nature photos to enjoy.
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 8 Views · 3 Likes
Flag
📝 Reply
Spectacular images, what wonderful places, the canyon is spectacular, unique. I would love to visit them in person.
Thanks for sharing, Dave.
Thanks for sharing, Dave.
▲
⟩⟩
Wolle
hermank
DWBrinkman
Login To
Remove Ads
Remove Ads
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 17 Views · 1 Like
Flag
📝 Reply
Hi Sir Kay, thanks for your reply.
Acrylic is another common term for PMMA. I wanted to be sure, and indeed, the Optix brand uses PMMA [or acrylic, if you prefer], which many know as Plexiglas because it's the most well-known brand.
This is what I've been able to determine from some online research.
Thank you for your kind words of appreciation.
Your display cases are excellent, beautiful, and functional. Yours aren't easy to manage, either; you've done a great job.
I've noticed that many companies create (even custom-made and made-to-order) frameless display cases; I think they're the norm.
They offer a better view and are aesthetically pleasing.
Acrylic is another common term for PMMA. I wanted to be sure, and indeed, the Optix brand uses PMMA [or acrylic, if you prefer], which many know as Plexiglas because it's the most well-known brand.
This is what I've been able to determine from some online research.
Thank you for your kind words of appreciation.
Your display cases are excellent, beautiful, and functional. Yours aren't easy to manage, either; you've done a great job.
I've noticed that many companies create (even custom-made and made-to-order) frameless display cases; I think they're the norm.
They offer a better view and are aesthetically pleasing.
▲
⟩⟩
hermank
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 19 Views · 1 Like
Flag
📝 Reply
Thank you so much Dave for the kind words, you are always very kind too.
▲
⟩⟩
hermank
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 22 Views · 2 Likes
Flag
📝 Reply
Thanks so much, Jumpugly, always such lovely words.
I've added more photos after your comment.
I've added more photos after your comment.
▲
⟩⟩
hermank
jumpugly
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 22 Views · 8 Likes
Flag
📝 Reply
...I have to attach the other images in this other post because I exceeded the maximum limit in the previous message.













































▲
⟩⟩
cenbeth
RNinMunich
hermank
JockScott
Mike Stoney
DWBrinkman
DuncanP
jumpugly
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
2 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 21 Views · 5 Likes
Flag
📝 Reply
Good morning everyone.
The ship model rests on a wooden base that is an integral part of the display case.
To access the model, you must lift the lid, which consists of a wooden frame and polycarbonate sheets.
Therefore, there are no doors or shutters.
The wooden base is made of a sheet of poplar plywood, approximately 20 mm thick.
The edges are made of four slats of fir wood (indicated by the red arrow), 20 mm thick and 10 mm wide, positioned like a frame.
This prevents the typical plywood layered edge from being visible.
The four fir strips are glued with vinyl glue but also have cylindrical reinforcement dowels (indicated by the yellow circles) that pass through (and are glued to) both the fir strips and the poplar plywood.
On this base are the supports to keep the ship model upright and stable.
Two Ayous wood strips (10 mm x 10 mm) (indicated by the green arrow) glued lengthwise to the aforementioned base and spaced apart serve to keep the ship upright by adhering (with a slight interlocking) to the keel.
If there is no particular stress, this is already sufficient to keep the ship upright.
However, there are eight additional supports for the same purpose.
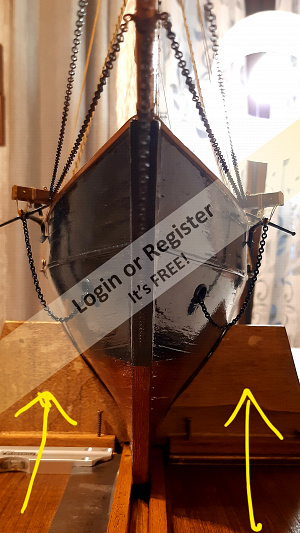
Four of them (indicated by the blue arrows) are lower and fixed, as they are glued to the wooden base.
Being low, they hold the hull well but do not obstruct the view.
The other four taller ones (indicated by the yellow arrows) are removable, in fact, they are fixed to the wooden base with screws. They are used when the display case is moved and can hold the ship in place even at a steep tilt (almost 90 degrees).
If the display case needs to be laid on its side for any reason (90-degree rotation), at least one strap or ribbon, which I haven't yet prepared, is needed to secure the ship.
The cover rests on the base just described.
The cover consists of four sides (two long and two short) made of transparent polycarbonate, through which the model can be seen. The roof is made of wood, while the base is, of course, empty.
This is the lid that rests on the base.
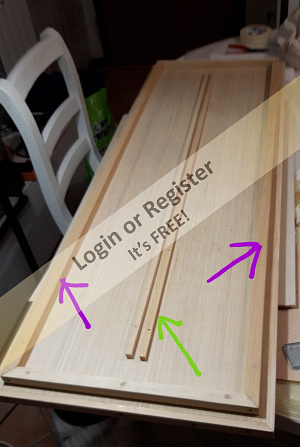
To prevent the lid from shifting, the base has an internal frame that fits inside the base of the lid (see purple arrows).
This internal rectangular frame on the base creates a slight interlocking, so the display case lid cannot shift, but can only be lifted.
To prevent it from being lifted, I added hooks (indicated by the orange arrows).
To transport the display case, you must grasp and lift it by the base.
To facilitate this operation, two handles can be screwed onto the short sides (see white arrow).
It took two people to build it.
It takes two people to transport it.
























The ship model rests on a wooden base that is an integral part of the display case.
To access the model, you must lift the lid, which consists of a wooden frame and polycarbonate sheets.
Therefore, there are no doors or shutters.
The wooden base is made of a sheet of poplar plywood, approximately 20 mm thick.
The edges are made of four slats of fir wood (indicated by the red arrow), 20 mm thick and 10 mm wide, positioned like a frame.
This prevents the typical plywood layered edge from being visible.
The four fir strips are glued with vinyl glue but also have cylindrical reinforcement dowels (indicated by the yellow circles) that pass through (and are glued to) both the fir strips and the poplar plywood.
On this base are the supports to keep the ship model upright and stable.
Two Ayous wood strips (10 mm x 10 mm) (indicated by the green arrow) glued lengthwise to the aforementioned base and spaced apart serve to keep the ship upright by adhering (with a slight interlocking) to the keel.
If there is no particular stress, this is already sufficient to keep the ship upright.
However, there are eight additional supports for the same purpose.
Four of them (indicated by the blue arrows) are lower and fixed, as they are glued to the wooden base.
Being low, they hold the hull well but do not obstruct the view.
The other four taller ones (indicated by the yellow arrows) are removable, in fact, they are fixed to the wooden base with screws. They are used when the display case is moved and can hold the ship in place even at a steep tilt (almost 90 degrees).
If the display case needs to be laid on its side for any reason (90-degree rotation), at least one strap or ribbon, which I haven't yet prepared, is needed to secure the ship.
The cover rests on the base just described.
The cover consists of four sides (two long and two short) made of transparent polycarbonate, through which the model can be seen. The roof is made of wood, while the base is, of course, empty.
This is the lid that rests on the base.
To prevent the lid from shifting, the base has an internal frame that fits inside the base of the lid (see purple arrows).
This internal rectangular frame on the base creates a slight interlocking, so the display case lid cannot shift, but can only be lifted.
To prevent it from being lifted, I added hooks (indicated by the orange arrows).
To transport the display case, you must grasp and lift it by the base.
To facilitate this operation, two handles can be screwed onto the short sides (see white arrow).
It took two people to build it.
It takes two people to transport it.

























▲
⟩⟩
cenbeth
RNinMunich
JockScott
DWBrinkman
jumpugly
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
3 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 21 Views · 0 Likes
Flag
📝 Reply
Hi Sir Kay, thanks for sharing your experience with so many helpful and informative details.
What are the dimensions of the display case you showed?
Do you remember the brand of clear acrylic used for your display cases?
What are the dimensions of the display case you showed?
Do you remember the brand of clear acrylic used for your display cases?
▲
⟩⟩
No likes yet
This member will receive 1 point
for every like received
This member will receive 1 point
for every like received
📝 Question of the Day?
3 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 40 Views · 5 Likes
Flag
📝 Reply
Yes, repetition is now physiological.
Let's look at it this way: new questions serve as a lesson, while repeated questions serve as a review.
Let's look at it this way: new questions serve as a lesson, while repeated questions serve as a review.
▲
⟩⟩
SouthportPat
Rockbag
SimpleSailor
DuncanP
Steves-s
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
3 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 29 Views · 0 Likes
Flag
📝 Reply
I agree, Jock. If I were to glue the sheets directly, I'd forgo the 45-degree cut and instead make it orthogonal just to have it lasered.
The corners of the parallelepiped might not look as nice as with the 45-degree joint, but they'll adhere well because they're lasered.
The corners of the parallelepiped might not look as nice as with the 45-degree joint, but they'll adhere well because they're lasered.
▲
⟩⟩
No likes yet
This member will receive 1 point
for every like received
This member will receive 1 point
for every like received
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
4 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 30 Views · 2 Likes
Flag
📝 Reply
Oh, okay, Jock, I didn't understand the question, sorry.
Now I do.
The specialized companies I contacted make display cases only with transparent sheets (PMMA or polycarbonate) without the need for a frame. Only one used a frame, but not a wooden one.
So let's say that display cases made entirely of PMMA (or similar) are the norm.
I must say, however, that the dimensions of my display case (especially the height) are a bit on the edge; in fact, someone said they could make it, but with a thickness of 5 mm instead of 4 mm.
When I asked for information about this on this same forum, they confirmed that it was possible.
I can tell you that the specific glues on the market guarantee a firm hold even over time; the problem is creating surfaces that fit together perfectly.
I hope I answered your question correctly this time, Jock.
Now I do.
The specialized companies I contacted make display cases only with transparent sheets (PMMA or polycarbonate) without the need for a frame. Only one used a frame, but not a wooden one.
So let's say that display cases made entirely of PMMA (or similar) are the norm.
I must say, however, that the dimensions of my display case (especially the height) are a bit on the edge; in fact, someone said they could make it, but with a thickness of 5 mm instead of 4 mm.
When I asked for information about this on this same forum, they confirmed that it was possible.
I can tell you that the specific glues on the market guarantee a firm hold even over time; the problem is creating surfaces that fit together perfectly.
I hope I answered your question correctly this time, Jock.
▲
⟩⟩
zooma
JockScott
📝 Animal and nature photos to enjoy.
4 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 18 Views · 3 Likes
Flag
📝 Reply
Hi Chris, your photos are truly splendid too.
Norway must be truly fantastic. Meanwhile, we Italians are also experiencing the current strength of their national football team.
Norway must be truly fantastic. Meanwhile, we Italians are also experiencing the current strength of their national football team.
▲
⟩⟩
ChrisF
hermank
Wolle
Login To
Remove Ads
Remove Ads
📝 Animal and nature photos to enjoy.
4 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 18 Views · 3 Likes
Flag
📝 Reply
Beautiful photos and beautiful places Dave, you are a great traveler and you do well.
▲
⟩⟩
DWBrinkman
hermank
Wolle
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
4 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 36 Views · 0 Likes
Flag
📝 Reply
I think so, Jock (but I hope I understand the question).
It depends on the size of the display case. In my case, the front wall was very tall and long, subject to a lot of flexing.
Without silicone (or other suitable glue) holding the wooden frame to the Lexan sheets, only the wooden frame will hold the structure together.
In my opinion, this is insufficient for these dimensions and with such thin boards (the grooves are shallow).
If someone thinks the display case should never be moved, then my whole point may seem superfluous. I assume the worst and believe the display case should also withstand movement (for cleaning, moving, etc.), even if limited or rare.
In this case (without glue), the stresses will only apply to the joints between the wooden boards.
If, however, there is glue in the grooves between the transparent sheets and the wooden boards, the display case becomes a single unit.
But, just out of curiosity, would you prefer not to use glue and just fit them into the grooves?
Are you already thinking about a display case for your tanker model?
It depends on the size of the display case. In my case, the front wall was very tall and long, subject to a lot of flexing.
Without silicone (or other suitable glue) holding the wooden frame to the Lexan sheets, only the wooden frame will hold the structure together.
In my opinion, this is insufficient for these dimensions and with such thin boards (the grooves are shallow).
If someone thinks the display case should never be moved, then my whole point may seem superfluous. I assume the worst and believe the display case should also withstand movement (for cleaning, moving, etc.), even if limited or rare.
In this case (without glue), the stresses will only apply to the joints between the wooden boards.
If, however, there is glue in the grooves between the transparent sheets and the wooden boards, the display case becomes a single unit.
But, just out of curiosity, would you prefer not to use glue and just fit them into the grooves?
Are you already thinking about a display case for your tanker model?
▲
⟩⟩
No likes yet
This member will receive 1 point
for every like received
This member will receive 1 point
for every like received
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
5 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 35 Views · 1 Like
Flag
📝 Reply
...now that I remember it had much better characteristics than Plexiglas, at least that's what I found written when I was researching the suitable material.
▲
⟩⟩
JockScott
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
5 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 35 Views · 1 Like
Flag
📝 Reply
Good evening everyone, before posting photos of the work, I need to make a correction.
The sheets are made of Lexan, a polycarbonate, not PMMA.
I carefully reviewed the various market research studies and, finally, the order.
The sheets are made of Lexan, a polycarbonate, not PMMA.
I carefully reviewed the various market research studies and, finally, the order.
▲
⟩⟩
JockScott
📝 Question of the Day?
5 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 48 Views · 1 Like
Flag
📝 Reply
Hi EdW, yes, indeed, after breathing helium you have a grotesque voice with a strange high-pitched tone.
Do you remember Buck and the others in the cartoon Ice Age?
I remember when we used to playfully inhale helium from inflatable balloons. We had ridiculous voices.
Do you remember Buck and the others in the cartoon Ice Age?
I remember when we used to playfully inhale helium from inflatable balloons. We had ridiculous voices.
▲
⟩⟩
Steves-s
📝 Question of the Day?
5 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 49 Views · 1 Like
Flag
📝 Reply
Hi EdW, if you can 3D print the design you posted, I'd really appreciate it.
As soon as you start working on it, I'll be happy to follow your lead.
As soon as you start working on it, I'll be happy to follow your lead.
▲
⟩⟩
Steves-s
📝 CONSTRUCTION OF A CASE FOR DISPLAYING A SCALE MODEL SHIP
6 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 56 Views · 2 Likes
Flag
📝 Reply
Hi Jock.
Yes, the frame is made of Samba wood (20 x 20 mm boards).
To join the wood, I used PVA glue with reinforcing pins.
The PMMA sheets are 4 mm thick; they are held together by grooves (as you can see in the photos and videos).
I put silicone in the grooves to glue the PMMA and wood (though I'm not sure if that was the best choice).
I didn't cut the PMMA myself; I ordered it directly with the measurements I needed, and I picked up the sheets ready-made.
I'll post photos of the construction as soon as I can.
Yes, the frame is made of Samba wood (20 x 20 mm boards).
To join the wood, I used PVA glue with reinforcing pins.
The PMMA sheets are 4 mm thick; they are held together by grooves (as you can see in the photos and videos).
I put silicone in the grooves to glue the PMMA and wood (though I'm not sure if that was the best choice).
I didn't cut the PMMA myself; I ordered it directly with the measurements I needed, and I picked up the sheets ready-made.
I'll post photos of the construction as soon as I can.
▲
⟩⟩
JockScott
jumpugly
📝 Question of the Day?
6 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 43 Views · 1 Like
Flag
📝 Reply
This was very easy for anyone who dives or has dived.
There wasn't even any need for translation; a few words like 100 meters were enough.
Plus, the question was only one of two options.
There wasn't even any need for translation; a few words like 100 meters were enough.
Plus, the question was only one of two options.
▲
⟩⟩
Steves-s
📝 Question of the Day?
6 days ago by 🇮🇹 AlessandroSPQR ( Fleet Admiral)
Fleet Admiral)
 Fleet Admiral)
Fleet Admiral)✧ 37 Views · 2 Likes
Flag
📝 Reply
Hi Steve, I think it's not impossible.
Very difficult, but doable.
As long as the scale isn't too small.
I also think many parts should be metal.
I don't have much faith in the durability and wear and tear of 3D printing materials.
The design is also doable, but certainly not easy.
If it's sold for £300, I suppose there's probably some quality to that part (including the materials used). Personally, it's not a device that excites me; I prefer traditional propellers.
I also have a lot of pending work and various electronic tests that I've neglected so much that I can't remember why I bought those individual components.
However, I'll be following with great interest anyone, like EdW, who has expressed interest in this type of propulsion system and who has the technical expertise to build it.
Very difficult, but doable.
As long as the scale isn't too small.
I also think many parts should be metal.
I don't have much faith in the durability and wear and tear of 3D printing materials.
The design is also doable, but certainly not easy.
If it's sold for £300, I suppose there's probably some quality to that part (including the materials used). Personally, it's not a device that excites me; I prefer traditional propellers.
I also have a lot of pending work and various electronic tests that I've neglected so much that I can't remember why I bought those individual components.
However, I'll be following with great interest anyone, like EdW, who has expressed interest in this type of propulsion system and who has the technical expertise to build it.
▲
⟩⟩
hermank
Steves-s





 Chief Petty Officer 2nd Class)
Chief Petty Officer 2nd Class)

 Lieutenant Commander)
Lieutenant Commander)

 Leading Seaman)
Leading Seaman)

 Commodore)
Commodore)

 Commander)
Commander)

 Captain)
Captain)


 Vice Admiral)
Vice Admiral)

 Rear Admiral)
Rear Admiral)

 Liked And...... another Titanic 20 hours ago
Liked And...... another Titanic 20 hours ago











